“Men of Galilee, why do you stand here looking into the sky?” — Acts 1:11
“Be patient, for the world is broad and wide.” — Romeo & Juliet
We are three-dimensional beings in a three-dimensional world. We have escaped our planet, but even then we traveled only in the dimensions we understand: height, length, and width. We can go up, down, left, right, back, and forward. Voyager 1, for example, has traveled 21 billion miles through this three-dimensional space. But always through one of three dimensions.
What if we could move in a new direction, along a new dimension? What if Jesus already has?
Scientists in string theory posit that there could be 10, 11, or 26 different spatial dimensions in our universe, depending on their particular scientific bent. It can be easy to dismiss those claims simply as theoretical or as too arcane to even matter; after all, most of us have a hard enough time navigating around our three dimensions without stubbing a toe or getting rear-ended by the tailgater behind us. What possible use could there be for a fourth dimension … or a tenth? What would any of that even look like? Shouldn’t it be impossible to even theorize a higher dimension’s attributes given our three-dimensional reality?
What if we could move in a new direction, along a new dimension?
Maybe not. Because we live in three dimensions, we have access to one- and two-dimensional reality as well. Let’s borrow an idea from Edwin Abbott’s satirical 1884 novella Flatland, in which points, lines, plane shapes, and a three-dimensional sphere think and interact with each other. Flatland is a world of two-dimensional shapes. We’d visualize them as squares, triangles, and other polygons, but to each other they appear simply as lines. They can’t see the whole shape in the same way that we can’t see an entire cube or globe at once—there’s always a “back side” we need rotation to see.
The main character of Flatland, A Square, visits Lineland to find that its inhabitants see each other only as points. To a Linelander, Square seems to appear and disappear suddenly as he passes through the kingdom, unable to convince its king that “besides your motion of Northward and Southward, there is another motion which I call from right to left.”
Similarly, A Square is visited by a sphere from Spaceland, who appears seemingly out of nowhere as a rapidly growing circle and introduces himself as “many circles in one.”
“I am in Space, and look down upon the insides of the things of which you only see the outsides,” the sphere explains. “A slight upward or downward motion would enable you to see all that I can see.” And with that, the sphere lifts the square up to view his world from that strange place—“above.”
From a mathematical standpoint, it would have been much more interesting had the visitor to Flatland not been a sphere, but a cone.
Imagine a cone-shaped light projecting from the ceiling to the floor of a room. The light is a single point on the ceiling, casting a two-dimensional circle on the floor. Now imagine you have a large sheet of poster board. If you inserted that paper into the cone of light perfectly parallel to the floor and ceiling, you would see a circle smaller than the one on the floor projected onto the paper.
Now, shift the poster board slightly so that it is not exactly parallel to the floor and the ceiling. The shape projected on the poster board is an ellipse. Tilt the poster board exactly parallel to the edge of the light cone, and you’ll get a parabola.
Conic sections (there’s one more: the hyperbola) are key to Euclidean geometry, and unpacking the awe and wonder they’ve prompted for mathematicians, astronomers, physicists, musicologists, paleontologists, and others would take us too far from our focus here. But two conclusions are worth lingering on: When measuring distances from fixed locations in three-dimensional space, the relationship is never linear. That is, you never get a straight line. Second, subtle changes in the three-dimensional world end up having a profound effect on the two-dimensional. Changing the angle of the poster board, for example, created a significantly different shape with significantly different mathematical properties. It doesn’t take much change in a higher dimension to have a big effect in a lower one.
This is where our most creative mathematicians and scientists have really started to make things interesting. Since we can study the relationship between the second and third dimensions, can we use our understanding of the third to predict what a fourth might look like? Can we “slice” a four-dimensional object in different ways and view its three-dimensional results?
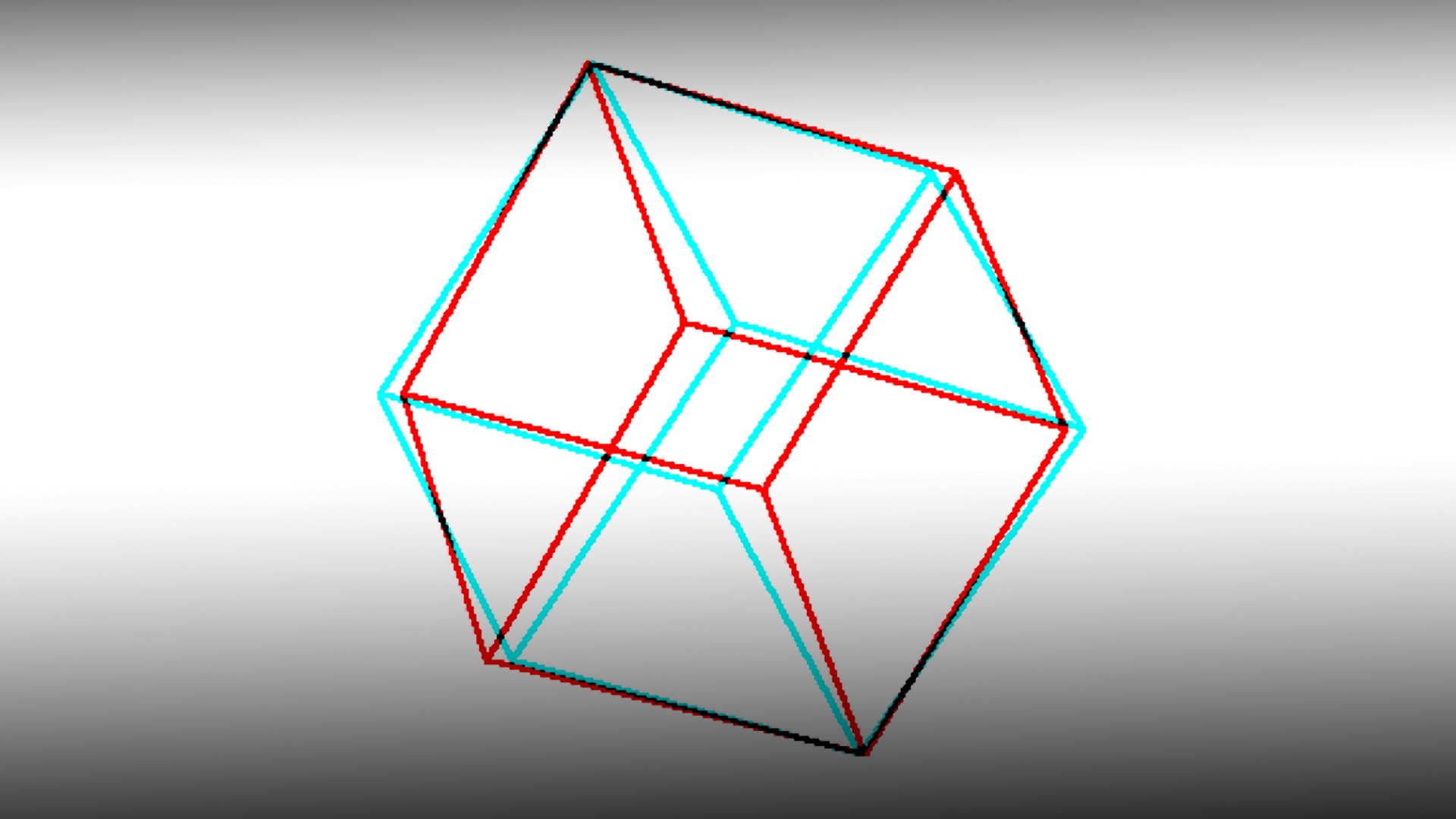
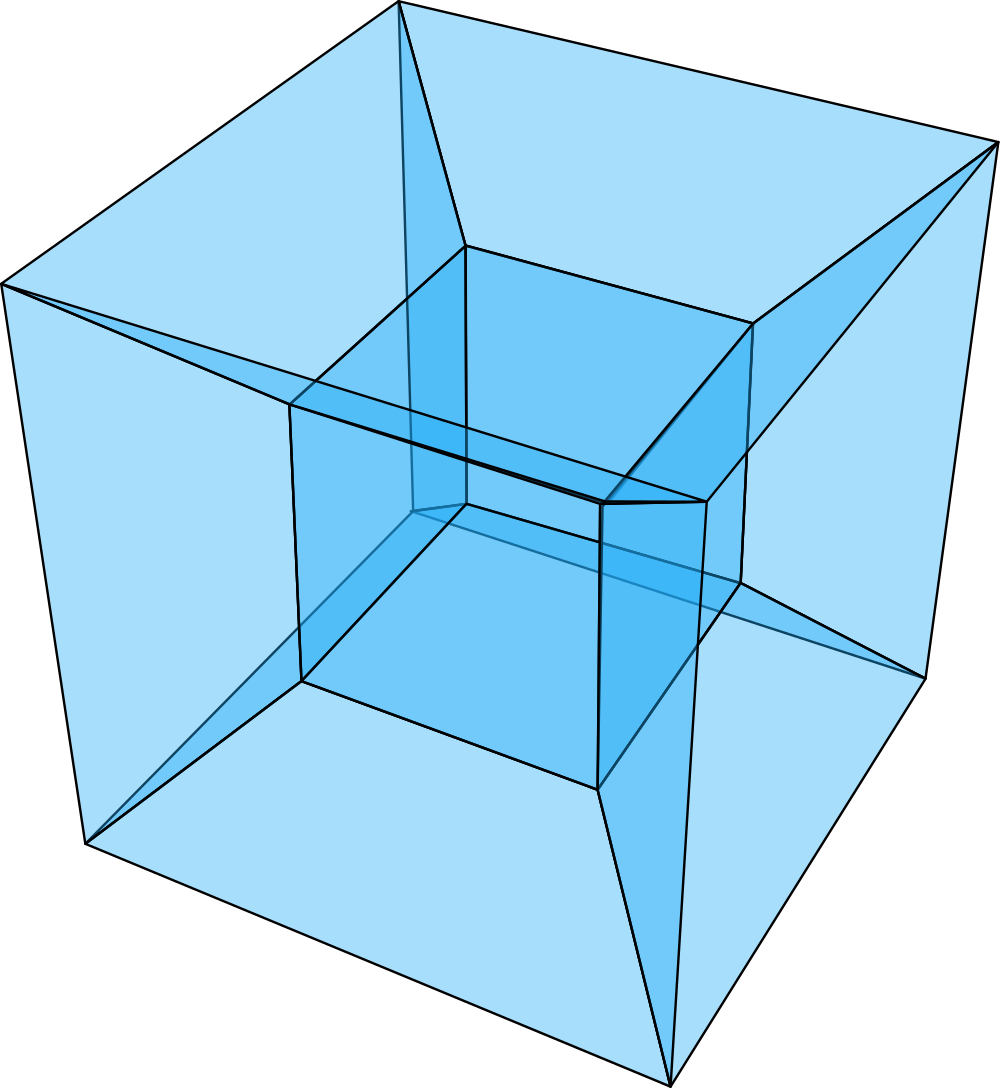
Yes, we can. At least, virtually. We can at least visualize it and suggest its shape like we can draw a cube on a piece of paper. Take, for example, the tesseract. Just like a cube is a three-dimensional object made up of two-dimensional squares, a tesseractis a four-dimensional object made up of three-dimensional cubes. We can’t build one. But we can kind of draw one:
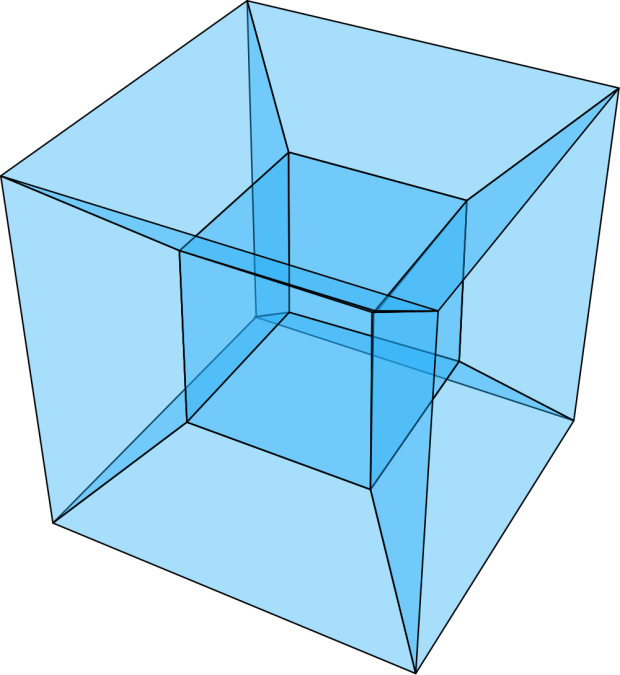
Just like a 3D cone can be “viewed” in 2D slices like circles, ellipses, parabolas, and hyperbolas, this is just one example of a tesseract “slice” in three dimensions—and then projected onto your two-dimensional screen. (You can play with other 3D slices and projections here.)
If we can create similar equations for 3D tesseract slices, we can attack problems with three unknowns instead of just two. Then maybe we can extend three to four. And four to five. And so on.
This is a big deal: Aren’t most things we encounter on a day-to-day basis affected by more than one or two conditions? Being able to analyze multiple dimensions allows for problem solving with more and more unknowns, which could lead to solutions for more difficult and obscure problems. Dimensional analysis (studying known dimensions in order to learn about higher ones) has been responsible for breakthroughs and discoveries in a variety of areas, including social networking analysis, medicine, security, and efficiency mapping.
But what if we could move in a new direction, along a new dimension?
And what if someone already has?
“Heaven is the extra dimension, the God-dimension, of all our present reality; and the God who lives there is present to us, present with us.” ~ N.T. Wright
We need to be careful here, because Scripture does not directly teach that heaven exists in other physical dimensions or that the resurrected Christ existed transdimensionally. But there are many indications in Scripture that could support this theory. The resurrected Jesus clearly existed both physically in a three-dimensional space (“Reach out your hand and put it into my side”) while transcending it (appearing suddenly in a locked room, disappearing at Emmaus, appearing without being recognized to loved ones).
If it’s true, it’s more than just some cool confluence of science and the Bible. As theologian N. T. Wright and others have suggested, it has radical implications for the way in which we view heaven, the dwelling place of God, how close God really is to us right now, and what’s really happening on Earth at this moment.
“The biblical notion of heaven is not of a place far away ‘way beyond the blue,’” Wright wrote. “Heaven is God’s space, which intersects with our space but transcends it. It is, if you like, a further dimension of our world, not a place far removed at one extreme of our world. … Heaven is the extra dimension, the God-dimension, of all our present reality; and the God who lives there is present to us, present with us, sharing our joys and our sorrows, longing as we are longing for the day when his whole creation, heaven and earth together, will perfectly reflect his love, his wisdom, his justice, and his peace. The ascension of Jesus, then, is his going, not way beyond the stars, but into this space, this dimension.”
Our hope is not simply to ascend into this new dimension when we die, but to see this world re-created in the multidimensional goodness, “on earth as it is in heaven.” Until then, we keep watch here in our three-dimensional Shadowlands. They may be miraculous, sudden, and impossible appearances, many circles in one, wheels within wheels. Or they may be simple ellipses on a plane, a bit of wine and bread, a loving touch in a time of need, a widow’s mite. On poster board and in our lives, the light still shines from above.
Joel Bezaire frequently writes on math for The Behemoth. Ted Olsen is editor of The Behemoth.